Constitutive model for elevated temperature flow stress of Ti–6Al–4V alloy considering the effect of work softening
-
摘要: 提出了一种包含流变软化的本构的构建方法。通过采用圆柱体试样的热压缩模拟试验，在塑性应变速率为0.1 s−1和20 s−1之间时，观察到TC4钛合金在750～950 ℃范围内均存在流变应力随着塑性应变降低的流变软化现象。采用双Voce方程对试验数据拟合得到了大塑性变形条件下的稳定流变应力。采用Levenberg-Marquardt非线性拟合算法得到了TC4钛合金包含流变软化的本构方程。并且发现Levenberg-Marquardt非线性拟合算法求得的本构方程参数比线性拟合误差更小。结果表明文中提出的流变应力计算方法规避了变形不稳定区域对特征变形抗力判断的干扰，得到了符合指数函数的材料高温稳定流变本构模型，在新型金属材料热加工工艺开发中具有较强的应用价值。Abstract: A method for establishing the constitutive model considering the flow softening was proposed. Isothermal uniaxial compression tests were performed on cylindrical specimens of Ti-6Al-4V at 750 ℃ to 950 ℃ and the strain rate of 0.1 s−1to 20 s−1. And the flow softening that the flow stress decreases with the plastic deformation was observed. The steady flow stresses under the severe plastic deformation were obtained by fitting the experimental data via double Voce function. The constitutive equation of the alloy considering the flow softening was acquired using Levenberg-Marquardt non-linear fitting. The parameters of the constitutive equation by Levenberg-Marquardt non-linear fitting have less error than those by the linear least square fitting. The proposed method for obtaining flow stress at high temperatures is able to avoid the influence of stress fluctuation in the early stage of hot deformation, and the high-temperature steady flow stress constitutive model according to the exponential function can be obtained, which will be useful in hot working process development for new metallic materials.
-
Key words:
- Ti-6Al-4V /
- flow softening /
- constitutive equation /
- thermocompression simulation
-
图 4 测量值和Voce函数拟合值的比较
Figure 4. Comparison between the measured and fitted stress-strain curves
图 5 稳定流变应力的样条曲面拟合
Figure 5. Bivariate spline approximation for the saturated flow stress
-
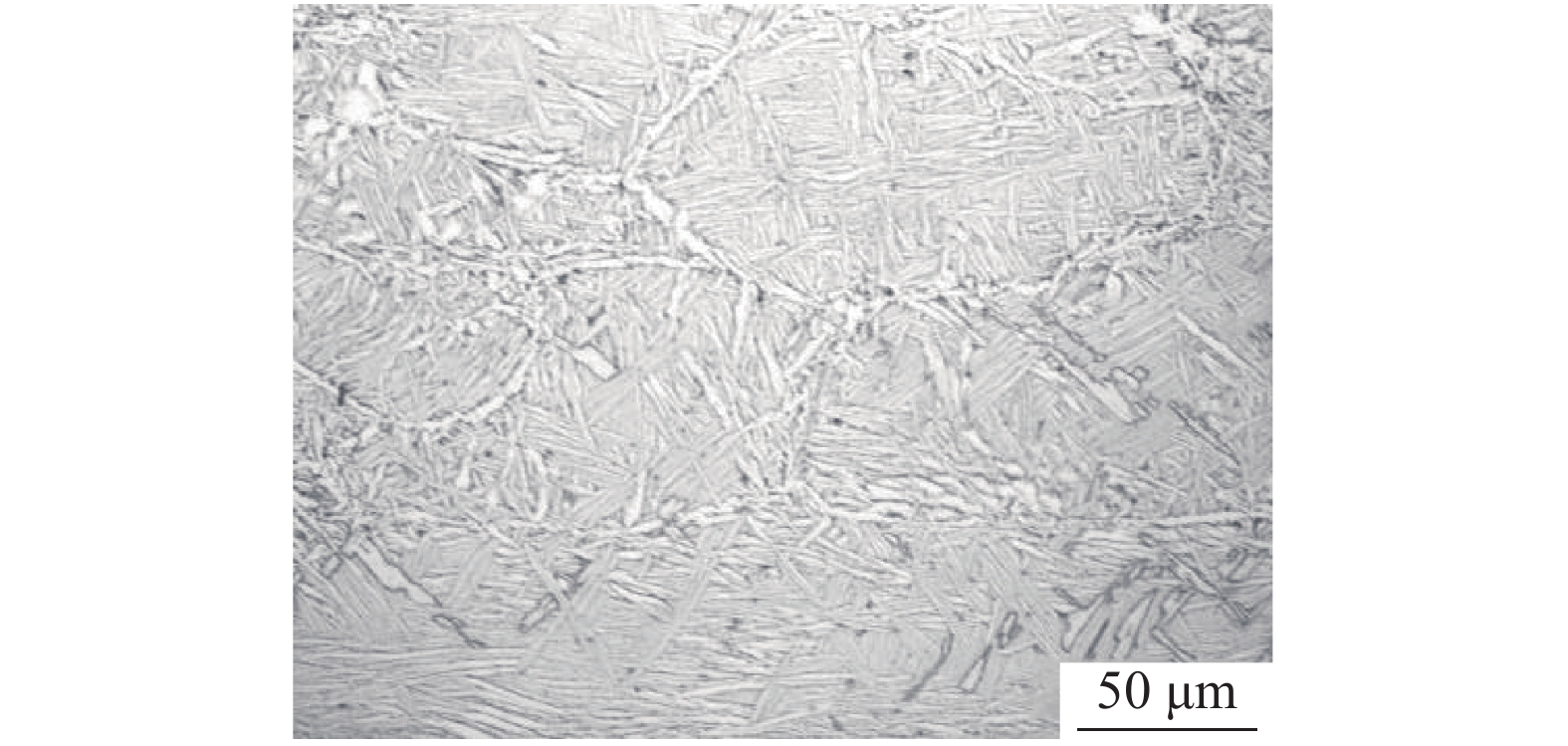
[1] Rugg David, Dixon Mark, Burrows Justin. High-temperature application of titanium alloys in gas turbines[J]//Material Life Cycle Opportunities and Threats – an Industrial Perspective. Materials at High Temperatures, 2016, 33 (4−5): 536−541. [2] Park Chan Hee, Kim Jeoung Han, Hyun Yong-Taek, et al. The origins of flow softening during high-temperature deformation of a Ti–6Al–4V alloy with a lamellar microstructure[J]. Journal of Alloys and Compounds, 2014,582:126−129. doi: 10.1016/j.jallcom.2013.08.041 [3] Bai Q, Lin J, Dean T A, et al. Modelling of dominant softening mechanisms for Ti-6Al-4V in steady state hot forming conditions[J]. Materials Science and Engineering: A, 2013,559:352−358. doi: 10.1016/j.msea.2012.08.110 [4] Alabort E, Putman D, Reed R C. Superplasticity in Ti–6Al–4V: Characterisation, modelling and applications[J]. Acta Materialia, 2015,95:428−442. doi: 10.1016/j.actamat.2015.04.056 [5] Lu Jiapeng, Chen Jianbin, Fang Qihong, et al. Finite element simulation for Ti-6Al-4V alloy deformation near the exit of orthogonal cutting[J]. The International Journal of Advanced Manufacturing Technology, 2016,85(9−12):2377−2388. doi: 10.1007/s00170-015-8077-z [6] Bennett, Christopher J, Sun Wei. Optimisation of material properties for the modelling of large deformation manufacturing processes using a finite element model of the gleeble compression test[J]. The Journal of Strain Analysis for Engineering Design, 2014,49(6):429−436. doi: 10.1177/0309324713520310 [7] Carsi Manuel, Bartolome M Jesús, Rieiro Ignacio, et al. The effect of heterogeneous deformation on the hot deformation of WE54 magnesium alloy[J]. Materials & Design, 2014,58:30−35. [8] Hu H E, Wang X Y, Deng L. Comparative study of hot-processing maps for 6061 aluminium alloy constructed from power constitutive equation and hyperbolic sine constitutive Equation[J]. Materials Science and Technology, 2014,30(11):1321−1327. doi: 10.1179/1743284714Y.0000000569 [9] Li X W, Lu M X, Sha Ai X, et al. The tensile deformation behavior of Ti–3Al–4.5V–5Mo titanium alloy[J]. Materials Science and Engineering: A, 2008,490(1−2):193−197. doi: 10.1016/j.msea.2008.01.086 [10] Gronostajski Z. The constitutive equations for FEM analysis[J]. Journal of Materials Processing Technology, 2000,106(1−3):40−44. doi: 10.1016/S0924-0136(00)00635-X [11] Gan Chunlei, Zheng Kaihong, Qi Wenjun, et al. Constitutive equations for high temperature flow stress prediction of 6063 Al alloy considering compensation of strain[J]. Transactions of Nonferrous Metals Society of China, 2014,24(11):3486−3491. doi: 10.1016/S1003-6326(14)63492-0 [12] Moré Jorge J. The levenberg-marquardt algorithm: Implementation and theory[M]//Numerical Analysis. Springer, 1978: 105−116. -
下载:
点击查看大图
图(7)
计量
- 文章访问数: 501
- HTML全文浏览量: 72
- PDF下载量: 52
- 被引次数: 0
